The following is an edited version of the interview.

EduNation: What do you see as the distinguishing feature of "Singapore Maths"? What makes it special?

Lee: It depends on who is looking at it. Every curriculum has so many different aspects. If you look at it simply from a Maths curriculum point of view, what captured the attention of the world was the structure of the curriculum. Our curriculum has a coherent framework that succinctly articulates our philosophy and beliefs of what we should do in a Maths classroom It is a holistic package that has key pedagogical approaches and key curriculum development approaches articulated in the curriculum. For example, the framework tells teachers what to emphasise in the classroom, while also describing how the content is developed to take into account the complexity of the discipline together with the cognitive development of the child. The pedagogical approach, especially at the primary level, advocates that teachers use a multiple representational approach, which we call "CPA" or "Cognitive-Pictorial-Abstract". Together, it's a comprehensive and holistic way of looking at curriculum. Over the years, we also take into account the deficiencies in our curriculum through curriculum review. But from my perspective, the biggest strength in our curriculum is that we do not rigidly follow a particular school of belief. Instead, we take an eclectic approach to the whole teaching and learning of mathematics.

EN: What kinds of schools of beliefs are there?

Lee: One school of belief is constructivism — they want learning to be very activity-based, whereas the old school of thought was behaviouristic — that mathematics requires a lot of practice. But if you look at Singapore Maths, we believe that there is a place for both in the curriculum. We look at the demands of the discipline or topic, and the skills that we would like to develop, and see what works best for our kids. This student-centric thinking in our curriculum is, to me, one of the biggest powers of our curriculum. Over time, the kind of student and the demands of the discipline will change. So the eclectic approach allows us to look at how different theories inform us in classroom teaching rather than our being driven by a theory.

Another thing about Singaporeans is that we are great travellers, even when it comes to education. People from the Ministry of Education and from NIE go out to the world, meet people and bring ideas back. But we don't just bring things back and push it into our curriculum; we will see how it fits into the bigger picture and make changes in a careful and calculated way. Education is one area where results or outcomes can only be seen many years later, and if we are too drastic in making changes, we will throw out the baby with the bathwater. So although we bring ideas back, we are very careful in the way we change things.

For example, Dr Kho Tek Hong, who was interviewed as part of an SG50 project about the development of the CPA approach, first went with his team to the US to research into learning theories. He learned about Bruner's⁵ ideas, made the theoretical ideas very concrete and changed it to the CPA approach, which is very much a Singapore Maths context. So you can see that we do learn from others, but we contextualise it, or I call it "operationalise" it, in the context of a classroom for teachers to implement.

EN: You mention the Singapore context; how is this different from that in other countries?

Lee: One example that is very distinct is that Singapore is a small nation. If we want to implement something, it's more manageable. For example, in Bhutan, where I was invited to act as consultant when they were reviewing their curriculum, they wanted to survey how lessons were conducted over the whole nation. Some of the villages were so far way that the data collectors had to travel for days, and hike up and down mountains and cross rivers. I was very amused when I heard that the surveyors needed to carry a sleeping bag, because they would have to find a cave to rest in and continue the journey the next day. And that was just for collecting data! If they wanted to call the teachers to come for training, it might not be impossible, but it would certainly not be as easy as in Singapore. Since it is easier for us to ensure that the average quality of classroom teaching is at a higher level across the whole nation, then it should not be surprising that the quality of the output would be higher

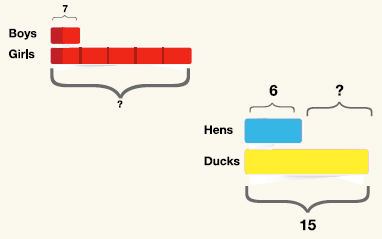The value placed on education is also very different. Ours is an Asian context, and Asians place a lot of value on education as a way to move out of poverty and up the social ladder. This may not be as much a value in some other countries. So the support students get from home in Singapore is more than students from some other parts of the world. In Singapore, even if the parents are not there, they will find some means to provide support for their children in completing their homework.

Our historical development has also had a large impact on our education. Without natural resources, our only resource was humans. The government has allocated nearly 12% of total government spending on education, higher than many other countries. Our classrooms are well-supplied with manipulatives and technological aids which promote more effective interaction in the classroom. So although we had to struggle in the years after independence, that has turned into our advantage. This kind of socio-cultural context can't be exported. This came with the history and the geography of our country.

EN: Let's talk about the bar model method, which has become the most well-known feature of Singapore Maths.

Lee: Once, I was invited to visit an international school that was using Singapore Math. I realised that solving every word problem started with the children drawing a bar model! I told them that it wasn't necessary, but the teacher said that it was their distinctive feature. I see the bar model method as one pedagogical tool which is a part of our package, but people like to focus on it because it looks unique. But a bar model is just a two-dimensional line diagram. Even before we used bar models, line diagrams were used to help students visualise the problems, but a two-dimensional diagram makes it more concrete for the young child. From my perspective, the introduction of the bar model has much pedagogical value. It was introduced at the primary level for good reasons: first of all, the bar model helps children by concretising the thinking in part-whole relationships, which is one of the keys in learning the four operations in arithmetic, whole numbers and fractions, and also basic arithmetic ideas. As children in primary school are still at the concrete operational stage, using something concrete makes it much easier for them to make sense of the concepts. So the bar model method lends itself very well to the kind of mathematics which primary students are working on.

Secondly, it is visual, it is a drawing. For young students, being concrete learners, the easiest way for them to solve a maths problem would be to draw or sketch. It also helps their memory because it taps on their sensory memory, and they can hold the pieces of information better when they write it down and draw it. So in terms of maths at the primary level, drawing models is one of the main heuristics for problem-solving.

I also think that it is wonderful that the bar model method also informally teaches our children algebraic thinking. In our current curriculum, lower secondary students do much more algebra than P5-P6 students. There is a big jump from very little formal algebra to a lot. I believe it was a conscious effort to bridge this gap by informally introducing algebraic thinking first. This is a very rich pedagogical tool — it meets the need of the discipline in terms of the part-whole thinking, it serves as a heuristic that students can employ to solve problems, and it develops informal algebraic thinking. On the other hand, sometimes in our enthusiasm, we can oversell. I think the ministry does try to alert teachers that they don't need to always use the model approach to solve everything. When used appropriately, it is powerful. If you overdo it, then it becomes a chore.

EN: Is maths regarded as "dry" and "forbidding"? Do teachers use different types of games in their curriculum?

Lee: We have been making conscious efforts to help students perceive maths differently. Now if you step into a primary maths class, it looks very colourful and cheerful compared with many years ago. Now there are colourful manipulatives. If you look at the primary maths textbooks, at the end of each chapter, there is always a game that students can play that is aligned with the concept they have just learned. I'm not too sure that students still think that maths is "dry" and "forbidding"?

What I'm really a little more concerned with is the change between maths in primary and secondary school. When primary school students become secondary school students, the textbooks and the style of teaching change. Secondary school maths is still pretty traditional. I think our secondary classrooms still await a reform.

EN: Do the various secondary school streams (Integrated Programme (IP), Express, Normal Academic (NA), Normal Technical (NT)) teach maths differently?

Lee: They do. In terms of catering to the needs of the various sections of students, I must say we have done a wonderful job.

If you look at the IP or gifted education programme, they basically stretch the students' mathematics in terms of depth and width. I think what is crucial is that the students have the opportunity to look beyond the curriculum without doing what I call acceleration. There are so many things that you can do with the main curriculum. We don't believe in acceleration for gifted kids in the Singapore context because the child may be very good in maths, but may not be as advanced in terms of social development. So these students stay in the same level and interact with others of their own age; it's just that they have been stretched through enrichment rather than acceleration.

At the same time, if you look at NT students, who tend to be more hands-on, teachers may trim down the curriculum in terms of cognitive demands, and try to do maths in a more hands-on way in the classroom. I think we have done very well in catering to the individual needs of the student.

But in terms of looking at the bulk of students (Express and NA) in secondary school, I find that secondary mathematics is still very traditional. I won't say it is not successful, but the approach is less broad. It is very teacher-centric instruction which targets a narrow band of students, but there are students with other needs. If we want to broaden our pedagogical approaches so we can reach out to more students, we will have to look for a slight "reform" of the secondary maths classroom for the bulk of the students, just like in primary schools in the late '80s.

EN: In Singapore, parents like to have their children "level up". If they know that there are some who are classified as "gifted" and given enrichment, they may pressure their children to challenge them. What is your opinion on this?

Lee: I think to every coin there are two sides. On the one hand, the value that parents place on education is really very useful in ensuring that our students are not deprived of a quality education. On the other hand, because of our parents's obsession with education, they may go the other way and overdo it. I do agree that there is a danger that this can escalate to the point that studying can become a chore.

I used to give talks to parents, and I would tell parents that sometimes, instead of encouraging our kids to love maths, we end up killing their interest. You can make it sound so formidable, as if it's really tough. I've seen parents, when their kids are preparing for the PSLE, buy a whole stack of exam papers for them. Imagine that you are a P6 kid and your parents give you a whole stack of exam papers for you to practise. I have asked parents, "If you know how to solve a problem, and you can solve it very well, then I give you 200 of these problems to solve. Do you think you can still solve them accurately and enthusiastically?"

Over the years, as our society talks more about stress in life and stress in school, and schools reach out more to parents, parents are also learning not to overdo things. One of the things I tell teachers is that parents have the same objectives as the teachers; they all want the child to do well. The difference between parents and teachers is that the teacher is trained pedagogically in how to teach the child while the parent is not. Another difference is that the teacher has 30-40 students to handle while the parent has only one. So if there is more communication between parents and teachers, where teachers share with parents the kind of things they can do at home, that will have impact and create a win-win situation for the child.

EN: What about students who are not in the gifted education programme (GEP), but who are actually strong in maths? How do schools cater to such children?

Lee: As far as I know, many schools have shared the materials which they use in GEP. There are efforts to integrate GEP students with the mainstream students, so there is a lot of sharing. Mainstream teachers are also equipped with the approach and the materials if they want to use them, and there is also an internet portal that teachers can access for shared lesson plans and ideas. I don't think it's a big issue.

The important point is how we can become a little more organised. Teachers are all very busy in the classroom, and we are all concerned with overall performance in exams and tests. Sometimes we get so bogged down with the middle portion of students that we forget that we have the few at the two ends. I always tell my student teachers that I have a 1-8-1 rule — no matter how homogenous our students are, there will always be differences between students. When I teach, I must recognise that I am likely to be able to cater instruction to the middle 80%. There will be the 10% who maybe already know what I am going to teach and would be interested in things beyond what I am teaching, and the other 10% who, no matter how hard I try, I cannot reach out to them yet because they need much more support. Many schools have remediation for some students from every class. I think there is also a need to have enrichment for some in every class. The question is: how can we plan school programmes such that those who need remediation are taken care of, and also those who need enrichment?

EN: Thank you for spending time to share these thoughts with us!